How to Calculate the Square Root: A Comprehensive Guide In 2024

Calculate the square root of a number is a fundamental mathematical operation that finds widespread use across various fields, including science, engineering, and everyday life. Whether you’re a student learning the basics of algebra or a professional needing to solve complex equations, understanding how to calculate the square root can be essential.

In this comprehensive guide, we will cover the concept of square roots, various methods for calculating square roots, and practical examples. We’ll also dive into why square roots are important and how you can calculate the square root using different techniques, whether manually or with the help of a calculator.

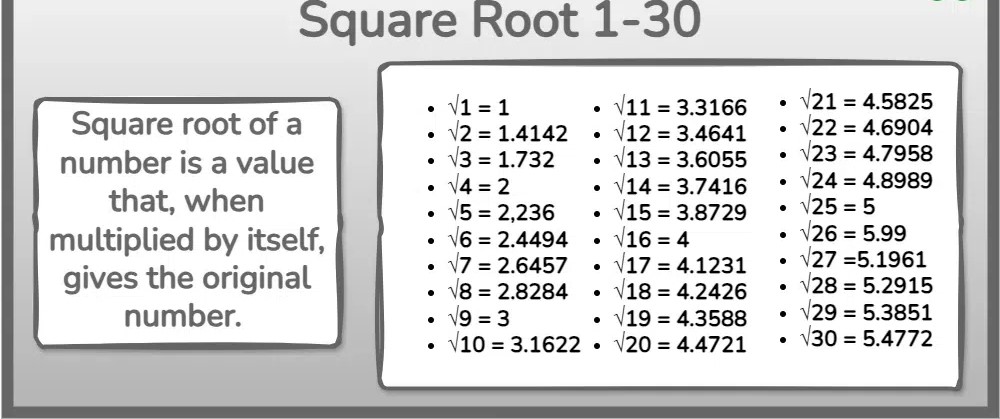
What is a Square Root?

Before diving into how to calculate the square root, let’s first understand what a square root is. The square root of a number xxx is a value yyy such that:y2=xy^2 = xy2=x

For example, the square root of 16 is 4 because 42=164^2 = 1642=16. In mathematical notation, the square root of xxx is written as x\sqrt{x}x​. The operation of finding the square root is essentially reversing the process of squaring a number.

Importance of Square Roots

Square roots are crucial in solving various types of mathematical problems. They are used in equations, geometric computations, physics, engineering, and finance. For instance, calculating the square root helps in finding the side of a square when the area is known, or in solving quadratic equations.

How to Calculate the Square Root

There are several methods to calculate the square root of a number. Let’s explore some of the most common techniques:

1. Using a Calculator

In today’s world, the simplest way to calculate the square root of any number is by using a calculator. Most scientific calculators have a dedicated button for square roots. You can calculate the square root in seconds by simply entering the number and pressing the square root key x\sqrt{x}x​.

Example:

To calculate the square root of 64 using a calculator, follow these steps:

1. Enter “64” on the calculator.
2. Press the x\sqrt{x}x​ button.
3. The result displayed will be “8,” since 64=8\sqrt{64} = 864​=8.

2. Manual Method – Prime Factorization

Another method to calculate the square root is through prime factorization. This method is particularly useful for perfect squares, numbers that have an exact square root. The steps for this method are:

1. Prime factorize the number by dividing it into prime numbers.
2. Group the prime factors in pairs.
3. Multiply one number from each pair to find the square root.

Example:

To calculate the square root of 144:

1. Prime factorize 144: 144=2×2×2×2×3×3144 = 2 \times 2 \times 2 \times 2 \times 3 \times 3144=2×2×2×2×3×3.
2. Group the prime factors: (2×2)(2 \times 2)(2×2) and (3×3)(3 \times 3)(3×3).
3. Multiply one number from each group: 2×3=122 \times 3 = 122×3=12.
4. Therefore, 144=12\sqrt{144} = 12144​=12.

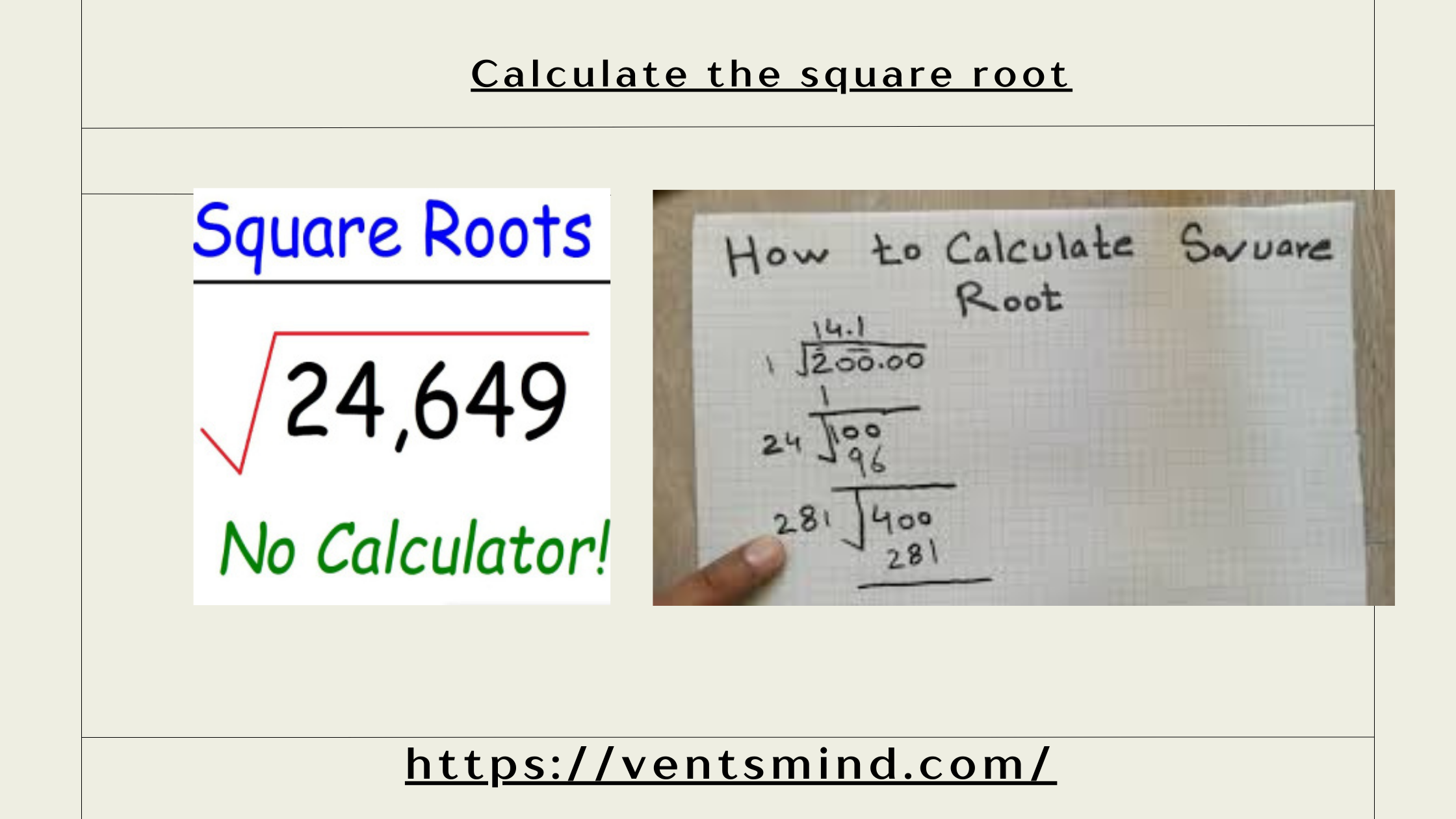
3. Long Division Method

The long division method is an old but reliable technique to calculate the square root of both perfect and non-perfect squares. This manual method can provide an accurate result up to several decimal places.

Steps to calculate the square root using long division:

1. Group the digits of the number into pairs, starting from the decimal point.
2. Find the largest integer whose square is less than or equal to the first pair.
3. Subtract the square of this integer from the first pair.
4. Bring down the next pair of digits.
5. Double the current quotient and find a number to append to it that forms the divisor for the next division.
6. Repeat the steps until you reach the desired accuracy.

Example:

To calculate the square root of 2025 using long division:

1. Group the digits of 2025 as 20 | 25.
2. Find the largest number whose square is less than or equal to 20 (in this case, 4).
3. Subtract 42=164^2 = 1642=16 from 20 to get 4.
4. Bring down the next pair (25) to make 425.
5. Double the quotient (4) to get 8. Now find a digit xxx such that 8x×x≤4258x \times x \leq 4258x×x≤425.
6. The answer is 5 because 85×5=42585 \times 5 = 42585×5=425.
7. Thus, 2025=45\sqrt{2025} = 452025​=45.

4. Estimation and Approximation

For non-perfect squares, calculating the square root often requires approximation. This method is especially useful when a calculator is unavailable, or you only need an approximate value. The process involves finding the nearest perfect squares and estimating the value between them.

Example:

To calculate the square root of 50:

1. Find the perfect squares around 50: 49=7\sqrt{49} = 749​=7 and 64=8\sqrt{64} = 864​=8.
2. Since 50 is closer to 49 than 64, estimate the square root to be slightly more than 7.
3. The approximation is around 7.07.

For a more precise calculation, you can use methods like Newton’s approximation.

5. Newton’s Method (Heron’s Method)

Newton’s Method, also known as Heron’s Method, is an iterative technique used to approximate square roots. The formula for this method is:xn+1=12(xn+Sxn)x_{n+1} = \frac{1}{2} \left( x_n + \frac{S}{x_n} \right)xn+1​=21​(xn​+xn​S​)

Where:

• SSS is the number you want to find the square root of.
• xnx_nxn​ is your current guess.

Example:

To calculate the square root of 10 using Newton’s Method:

1. Start with an initial guess, say 3.
2. Apply the formula: x1=12(3+103)≈3.1667x_1 = \frac{1}{2} \left( 3 + \frac{10}{3} \right) \approx 3.1667×1​=21​(3+310​)≈3.1667.
3. Repeat the process: x2=12(3.1667+103.1667)≈3.1623x_2 = \frac{1}{2} \left( 3.1667 + \frac{10}{3.1667} \right) \approx 3.1623×2​=21​(3.1667+3.166710​)≈3.1623.
4. Continue this process until the value converges.

Using Newton’s Method, you can calculate the square root to any desired level of accuracy.

Real-World Applications of Calculating the Square Root

Knowing how to calculate the square root is important in various practical situations. Here are a few examples where square root calculations are essential:

1. Geometry and Construction: In geometry, calculating the square root is crucial for determining the length of the diagonal of a square or the hypotenuse of a right triangle using the Pythagorean theorem.
2. Physics and Engineering: Square roots are frequently used to solve equations in physics, such as when calculating velocities, forces, and accelerations.
3. Finance: In finance, square roots are often used in risk analysis, particularly in calculating the standard deviation of investment returns.
4. Statistics: In statistics, square roots are fundamental in calculating standard deviations and variances, which are key in data analysis.

Conclusion

Understanding how to calculate the square root is a valuable skill in both academic and practical contexts. Whether you’re working with a perfect square, estimating a value, or using advanced methods like Newton’s Method, there are multiple ways to approach square root calculations. From the simplicity of using a calculator to the elegance of manual techniques like prime factorization and long division, mastering these methods equips you with a critical mathematical tool.

By practicing these techniques, you’ll be able to calculate the square root of any number, whether you’re solving for exact or approximate values. As you encounter more complex problems, this skill will prove invaluable across a range of disciplines, including geometry, physics, engineering, and finance.